Examples of Plane Shapes

PROPERTIES OF SOME PLANE SHAPES

• Rectangle:
  
  • Every interior angle of a rectangle is equal to 90°, i.e. ∠A = ∠B = ∠C = ∠D = 90°
  • The sum of the interior angles is equal to 360°.
  • The opposite sides are equal and parallel to one another, i.e. AB = CD & AC = BD.
  • The diagonals are congruent.
  • The diagonals bisect each other, i.e AO = OD & BO = OC.
  • The diagonals bisect each other at different angles. One of them is an acute angle, while the other is an obtuse angle.
  • Any diagonal of a rectangle is a diameter of its circumcircle.

• Square:
  
  • A square has 4 vertices and 4 sides.
  • Each of the interior angles is equal 90°, i.e. ∠A = ∠B = ∠C = ∠D = 90°.
  • The sum of the interior angles is equal to 360°.
  • Each side of a square is equal, i.e. AB = BD = CD = AC
  • The opposite sides of a square are parallel to each other.
  • The diagonals of a square are equal.
  • The diagonals of the square bisect each other at 90°.
  • The diagonal of a square divides it into two similar isosceles triangles.

• Circle:
  
  • Circles are said to be congruent if they have equal radii.
  • Equal chords and equal circles have equal circumference.
  • The diameter of a circle is the longest chord of a circle.
  • The radius drawn perpendicular to the chord bisects the chord.
  • Circles having different radii are similar.
  • A circle can circumscribe a rectangle, trapezium, triangle, square, kite.
  • A circle can be inscribed inside a square, triangle, and kite.
  • The chords that are equidistant from the center are equal in length.
  • The distance from the center of the circle to the longest chord (diameter) is zero.
  • The perpendicular distance from the center of the circle decreases when the length of the chord increases.
  • If the tangents are drawn at the end of the diameter, they are parallel to each other.
  • An isosceles triangle is formed when the radii joining the ends of a chord to the center of a circle.

• Kite:
  
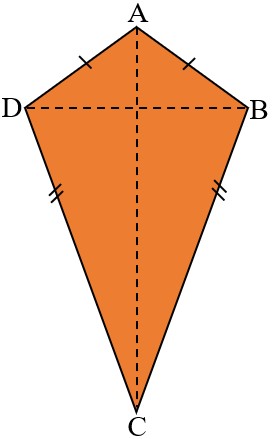
  • Two disjoint pairs of consecutive sides are congruent by definition, i.e. AB = AD and BC = CD.
  • The diagonals are perpendicular; they meet at right angles.
  • One diagonal (segment AC) is the perpendicular bisector of the other diagonal (segment BD)
  • The diagonal AC bisects a pair of opposite angles (angle A and angle C).
  • The opposite angles at the endpoints of the cross diagonal are congruent (angle D and angle B)

PERIMETER OF PLANE SHAPES

• The perimeter of a shape is a measure of the distance round the boundary or edge of the shape.
• With the usual lettering, the perimeter of a rectangle is 2(L + B), square is 4L, circle is 2πr or πd.
• The perimeter of a circle is called the circumference.

AREA OF PLANE SHAPES

• The area of a shape is a measure of its surface.
• Area is measured in units such as cm² or m², which are derived from the SI units of length.
• The areas of common plane shapes are as follows:
  • Rectangle = L × B
  • Square = L²
  • Parallelogram = Base × Height
  • Triangle = ½ × Base × Height
  • Trapezium = ½ h(a + b)
  • Circle = πr²

Examples

1. Calculate the perimeter of a football field which measures 80 m by 50 m.
   • Perimeter of field = 2(L + B)
   •                               = 2(80 + 50)
   •                               = 2 × 130
   • ∴ The perimeter of the field = 260 m
2. A rectangle has a perimeter of 74 m. Find
   1. the length of the rectangle if its breadth is 17 m.
      • Perimeter of a rectangle = 2(L + B)
      • Perimeter = 74 m,
      • Breadth = 17 m,
      • ∴ 74 = 2(L + 17)
      • ⁷⁴/₂ = L + 17
      • 37 = L + 17
      • L = 37 – 17
      • ∴ Length of the rectangle = 20 m.
   2. the breadth of the rectangle if its length is 25 m.
      • Perimeter of a rectangle = 2(L + B)
      • Perimeter = 74 m,
      • Length = 25 m,
      • ∴ 74 = 2(25 + B)
      • ⁷⁴/₂ = 25 + B
      • 37 = 25 + B
      • B = 37 – 25
      • ∴ Breadth of the rectangle = 12 m.
3. Calculate the perimeter of a square of side 12.3 cm.
   • Perimeter of a square = 4L,
   • L = 12.3 cm,
   • ∴ Perimeter = 4 × 12.3
   • ∴ Perimeter of the square = 49.2 cm.
4. A square lawn has a perimeter of 56 m. Find the length of the side of the lawn.
   • Perimeter of a square = 4L,
   • Perimeter = 56 m,
   • ∴ Length of the side = ⁵⁶/₄
   • ∴ Length of the side of the lawn = 14 m.
5. Calculate the circumference of a circle of radius 3¹/₂ m. (Let π = 3¹/₇).
   • Circumference of a circle = 2πr,
   • R = 3¹/₂ ,
   • ∴ Circumference of a circle = 2 × 3¹/₇ × 3¹/₂
   •                                                = 2 × ²²/₇ × ⁷/₂
   •                                                = 2 × 11
   • ∴ Circumference of a circle = 22 m
6. A bicycle wheel has a diameter of 65 cm. During a journey, the wheel makes 1000 complete revolutions. How many meters does the bicycle travel? (Let π = 3.14)
   • Circumference of a circle = πd,
   • Distance traveled in one revolution = 3.14 × 65 cm
   • Distance traveled in 1000 revolutions = ^{(3.14 × 65 × 1000 m)}/₁₀₀
   • ∴ Distance traveled in 1000 revolutions = 31.4 × 65 m
   • ∴ Distance traveled in 1000 revolutions = 2041 m
7. Calculate the area of a rectangle 6 cm by 3.5 cm.
   • Area of a rectangle = L × B
   • L = 6 cm
   • B = 3.5 cm
   • ∴ The area of the rectangle = 6 × 3.5
   • ∴ The area of the rectangle = 21 cm²
8. The area of a rectangle is 224 cm². If its length is 16 cm, calculate the breadth.
   • Area of a rectangle = L × B
   • Area = 224 cm²
   • L = 16 cm
   • ∴ 224 = 16 × B
   • B = ²²⁴/₁₆
   • ∴ Breadth of the rectangle = 14 cm²
9. The area of a square plot is 144 m². Calculate the length of a side of the plot.
   • Area of a square = L²
   • Area = 144 m²
   • ∴ 144 = L²
   • ∴ L = √144
   • ∴ L = 12 m
10. An assembly area is in the shape of a 30 m by 30 m square. Part of the area is a concrete rectangle 25 m by 5m, the rest is grass. Calculate the area of the grass.
    
    • Area of assembly area = L²
    •                                          = 30²
    • ∴ Area of assembly area = 900 m²
    • Area of concrete = L × B
    •                                = 25 × 5
    • ∴ Area of concrete = 125 m²
    • ∴ Area of grass = 900 – 125
    • ∴ Area of grass = 775 m²
11. Calculate the area of a parallelogram if its base is 9.2 cm and its height is 6 cm.
    • Area of parallelogram = base × height
    •                                            = 9.2 × 6
    • ∴ Area of the Parallelogram = 55.2 cm²
12. In the figure below, the base of the parallelogram is 6 cm and its height is 4 cm. Calculate the area of the parallelogram. If the length of the other side of the parallelogram is 8 cm, calculate the corresponding height, h.
    
    • Area of parallelogram = 6 × 4
    • ∴ The area of parallelogram = 24 cm²
    • Also, the area of the parallelogram = 8 × h
    • ∴ 24 = 8 × h
    • ∴ h = ²⁴/₈
    • ∴ The height = 3 cm
13. Calculate the area of the triangle shown in the figure below.
    
    • Area of a triangle = ½ × B × H
    •                                      = ½ × 9 × 12
    • ∴ The area of the triangle = 54 cm²
14. In the diagram below, the diagonal AC divides the trapezium into two triangles. The height of each triangle is 8 cm. Calculate the area of the trapezium.
    
    • Area of Δ ACB = ½ × 13 × 8 = 52 cm²
    • Area of Δ ACB = ½ × 6 × 8 = 24 cm²
    • Area of trapezium = Area of Δ ACB + Area of Δ ACB
    •                                      = 52 + 24
    • ∴ The area of the trapezium = 76 cm²
15. Find the area of a circle of radius 3¹/₂ meters. (Let π = ²²/₇)
    • Area of a circle = πr²
    •                              = ²²/₇ × (3¹/₂)²
    •                              = ²²/₇ × ⁷/₂ × ⁷/₂
    •                              = ¹¹/₂ × 7
    •                              = ⁷⁷/₂
    • ∴ The area of the circle = 38¹/₂ m²
16. Calculate the area of the shape in the figure below. (Let π = ²²/₇). The radius of the semi-circle is 7 m.
    
    • Area of shape = Area of Big Rectangle + Area of Small Rectangle + Area of Semi Circle
    • Area of big rectangle = 16 × 10 = 160 m²
    • Area of small rectangle = 14 × 1 = 14 m²
    • Area of semi circle = ½ × ²²/₇ × 7 × 7 = 77 m²
    • ∴ The area of the shape = 160 + 14 + 77
    • ∴ The area of the shape = 251 m²